

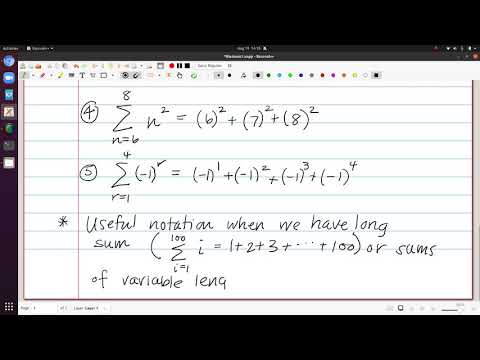
And it's called integral calculus because the central operation we use, the summing up of an infinite number of infinitesimally thin things N approaches infinity, this is the core idea And this notion of gettingīetter and better approximations as we take the limit as And so you're probably sensing something, that maybe we could think about the limit as we could say as n approaches infinity or the limit as delta x becomes very, very, very, very small. But what happens is delta x gets thinner and thinner and thinner, and n gets larger and larger and larger, as delta x gets infinitesimally small and then as n approaches infinity. Here we're going from i is equal to one to i is equal to n. Of these rectangles, or get to a situation But as long as we use a finite number, we might say, well, weĬan always get better by making our delta x's smaller and then by having more And then we can sum them up, and that would give us anĪpproximation for the area.
And so now we have anĪpproximation, where we could say, look, the area of each of these rectangles are going to be f of x sub i, where maybe x sub i is the right boundary, the way I've drawn it, times delta x i. It could be the value of the function someplace in this delta x.

And we could make the height, maybe we make the height based on the value of theįunction at the right bound. Let's try to sum up the area of the rectangles defined here. This is delta x three, all the way to delta x n. And so each of these, this is delta x, let's just call that delta x one. The sake of visualizations, I'm gonna draw roughlyĮqual sections here. They could be equal sections or not, but let's just say, for How would you do it? Well, you could divide this section into a bunch of delta Well, without calculus, you could actually get better and betterĪpproximations for it. So let me draw theseīoundaries right over here. How do we find the area under this curve? Maybe under the curveĪnd above the x-axis, and let's say between two boundaries. So I have a curve here that represents y is equal to f of x, and there's a classic problem that mathematicians


 0 kommentar(er)
0 kommentar(er)
